math
Hazard to Others
  
Posts: 101
Registered: 21-7-2006
Member Is Offline
Mood: No Mood
|
|
Iron bar bending force (physics question)
hello everyone,
I've got this physics related problem banging in my head and I don't know how it could be calculated/answered.
Given an iron bar, of round cross-section, fixed by its extremities
(bar measures 152 mm in length and 4.8 mm in diameter),
a weight of 88.5 kgs is hung from its middle, bending it at least
90 degrees.
How much weight/force would be needed to bend another
bar of the same material and size, but of hexagonal or square
section?
I guess the answer relies on calculus skills I'm not aware or lacking of  , maybe
that's why I find this real-life problem that interesting , maybe
that's why I find this real-life problem that interesting  . .
Thank you all in advance
|
|
|
ScienceGeek
Hazard to Others
  
Posts: 151
Registered: 22-1-2008
Location: Norway
Member Is Offline
|
|
I hate myself right now! I just HAD to read this question, and now as I don't have an answer to it, it will be bugging me until someone is able to
answer it.
It is a very good question, nonetheless. Very good indeed. I am not sure you need calculus to solve it, though. I can't think of any way that you
would...
Structurally, a square or hexagonal bar would have different properties compared to a round one. As you bend it, the material will compress and
stretch differently at different "corners", of which I have no idea how to calculate.
Also, a square bar would behave quite differently from a hexagonally formed bar because the hexagonal bar allows itself to be compressed at the point
of bending more easily than the square one. This is because of the shape of a hexagon.
I hope someone is able to figure this one out!
Sorry I couldn't help you more than this 
|
|
|
math
Hazard to Others
  
Posts: 101
Registered: 21-7-2006
Member Is Offline
Mood: No Mood
|
|
Thank you for spending some time to reply 
yes actually the only real life-supposition I can do
is that a bar of same size and material, is more
likely to bend the more "sides" its cross-section has.
For example a round bar takes less force to bend than
an hexagonal one, and in turn that bar takes less force
to bend than a square one...with the above list
ending with a bar of triangular cross-section.
Hope some guru will enlighten us on this subject 
|
|
|
Magpie
lab constructor
    
Posts: 5939
Registered: 1-11-2003
Location: USA
Member Is Offline
Mood: Chemistry: the subtle science.
|
|
I can't solve this problem but remember from my strength of materials class that a pipe of the same unit mass is much stronger than a rod of the same
unit mass, in bending.
I suspect that the math required to prove the answers to your question would be quite complex and difficult.
|
|
|
12AX7
Post Harlot
    
Posts: 4803
Registered: 8-3-2005
Location: oscillating
Member Is Offline
Mood: informative
|
|
The question is not nearly well enough specified. What are the properties of this iron bar? "Iron" often refers to gray cast iron, which doesn't
bend at all. Some alloys (admittedly, none(?) of them iron alloys) can be bent much tighter than this while in elastic deformation. Pure, annealed
iron will undergo considerable plastic deformation, while spring hard chromium steel will retain considerable elastic force (probably retaining some
plastic deformation).
This question does not specify if the endpoints are allowed to slide or if the bar is rigidly clamped in place. If so, it must be stretching a
considerable amount -- at least 40%, which is more ductile than most alloys!
If the problem were well specified, the complete analytical answer lies somewhere in tensor calculus, because stress and strain can be expressed by a
tensor. Some tensors here:
http://mysite.du.edu/~jcalvert/phys/physhom.htm
Tim
|
|
|
pantone159
National Hazard
   
Posts: 586
Registered: 27-6-2006
Location: Austin, TX, USA
Member Is Offline
Mood: desperate for shade
|
|
I think the bending involved is so large that permanent deformation is occurring, which makes things 1) far more complicated, and 2) probably
incompletely specified.
When elastic deformation is involved, there are beam equations that can be used, differential equations of some kind, I forget, although I did take a
class in this. (Kind of fun math.) If you are willing to change 'at least 90 degrees' to 'at least 0.9 degrees' then you can make progress.
|
|
|
math
Hazard to Others
  
Posts: 101
Registered: 21-7-2006
Member Is Offline
Mood: No Mood
|
|
thank you for your points 12AXT.
Actually, the question is quite specific and abstract, as it doesn't consider material specifically, costants etc, but rather the DIFFERENCE in
bending forces between two bars of same material and size but different cross-section shape, given the strength of a round one;
so it would have been the same if we'd have considered aluminum bars, zinc bars etc...I wouldn't point out this material issue that much.
Well to be realistic, yes, consider the bar sliding and not stretching of course 
Thanks to a friend of mine, I've got two real-life info about iron bar bending (allegedly middle-steel), they could be used to make a percentage
proportion, still usable but not as satisfying as the answer we're searching for:
152 x 4.8mm round section (61.3 kg to bend)
152 x 4.8mm square section (95.3 kg to bend)
178 x 6.4mm round section (108.9 kg to bend)
178 x 6.4mm square section (172.4 kg to bend)
|
|
|
pantone159
National Hazard
   
Posts: 586
Registered: 27-6-2006
Location: Austin, TX, USA
Member Is Offline
Mood: desperate for shade
|
|
| Quote: | Originally posted by math
Actually, the question is quite specific and abstract, as it doesn't consider material specifically, costants etc, but rather the DIFFERENCE in
bending forces between two bars of same material and size but different cross-section shape, given the strength of a round one;
so it would have been the same if we'd have considered aluminum bars, zinc bars etc...I wouldn't point out this material issue that much.
|
For such a large amount of bending, the specific material will be very important and not neglectable. You are past the point where the material
behaves like a spring and the crystal structure is being permanently changed, and the particular crystal involved will matter very much.
Are you really interested in such a large amount of deformation? That vastly complicates your question.
|
|
|
The_Davster
A pnictogen
      
Posts: 2861
Registered: 18-11-2003
Member Is Offline
Mood: .
|
|
I think that the particular material may be irrelevant depending on the exact focus of the question. I am not well versed in engineering, but it
seems like there would be two equations for solid vs tubular, where the metal identity's property would be a constant in each equation, and when
comparing the two that these would cancel out making the exact identity of the metal irrelevant.
Kind of like a physics question in which an object falls to the ground from a certain height in a certain time, find the time it takes to hit the
ground from another height, in which the mass of the object is constant between the two, so one does not need to know it.
Of course the metal bar question is going to involve some much more heavy duty math than my example.
|
|
|
tumadre
Hazard to Others
  
Posts: 171
Registered: 10-5-2005
Member Is Offline
Mood: No Mood
|
|
The material properties matter in this case, because if you notice, the inside edges of a square/rectangular bar can bend outward in permanent
deformation, while the bulk of the bar remains elastic and or plastic.
I have never seen this with a round bar.
if you were to bend the bar back straight, maybe repeat once more, most alloys will break along this inside corner.
but some aluminum alloys will break along the outside, when bending to 90degrees the second time.
A while back a friend of mine and i concluded this is best left to empirical formulas, even though we had access to matlab and a few "educated"
professors.
edit:
152 x 4.8mm round section (61.3 kg to bend)
152 x 4.8mm square section (95.3 kg to bend)
178 x 6.4mm round section (108.9 kg to bend)
178 x 6.4mm square section (172.4 kg to bend)
I find it interesting, that the ratio between the two forces is very close to the ratio of cross sectional area squared. .785^2 = .616
[Edited on 15-6-2008 by tumadre]
|
|
|
JohnWW
International Hazard
    
Posts: 2849
Registered: 27-7-2004
Location: New Zealand
Member Is Offline
Mood: No Mood
|
|
For a start, this problem is one of "squaring the circle"! Firstly, one has to calculate the lengths of the cross-sections of each side of a square
and of a hexagon with the same cross-sectional area as the circular-sectioned bar. These are simple exercises in geometry.
But after that, the fun starts. One has to calculate the stress required for the permanent deformation of the circular bar, which is reasonably
straightforward from the standard formula for the bending moment. The required stress on bending a square-sectioned bar of the same material can then
be calculated from the bending-moment formula for a square bar, although it is not stated whether the bending of it is parallel to two of the faces or
diagonally, for which there are two different mending-moment formulas. There must be similar bending-moment formulas somewhere for the bending of a
hexagonal bar either at right angles to two faces or diagonally. References such as the "Mechanical Engineer's Handbook", "Mechanical Engineering
Handbook", "Mechanical Engineer's Reference Book", and "Machinery's Handbook" should have these formulas; I will see about finding uploads of them, or
uploading them for the References section.
[Edited on 16-6-08 by JohnWW]
|
|
|
franklyn
International Hazard
    
Posts: 3026
Registered: 30-5-2006
Location: Da Big Apple
Member Is Offline
Mood: No Mood
|
|
This is not a hard question, I'm sure there are standard engineering formulas that can be
applied. The answer will vary depending on the orientation of the bar to the applied
bending force. It's been to long since I took strength of materials to be of further help.
You may also try posting to an appropriate forum here _ http://www.eng-tips.com
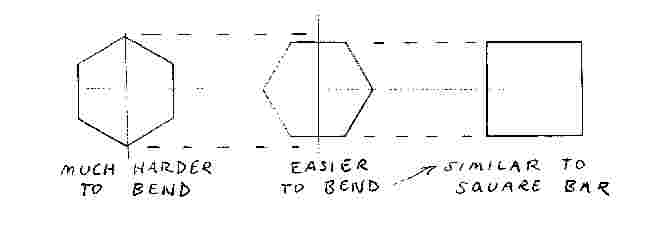
|
|
|
Geomancer
Hazard to Others
  
Posts: 228
Registered: 21-12-2003
Member Is Offline
Mood: No Mood
|
|
[NB: I started writing this last evening, before many of the posts now present were made. Those posts address many of the same issues.]
Generally when materials deform continuously under force they are assumed to behave in a certain way. Up to a point (the "elastic limit") they
behave as springs: the amount they stretch is reversible and directly proportional to the strength of the force. This is the "elastic deformation"
12AX7 was referring to. Beyond the elastic limit they continue to deform, however the amount of force doesn't increase (ideally), and the deformation
is not reversible ("plastic deformation"). So, as 12AX7 noted, the nature of your solution will depend on to what degree you exceed the elastic limit
of your material. If, upon removing the load, the beam springs back to its original shape then you're in luck: you can be certain the deformation was
entirely elastic.
One can easily calculate the degree of bending in a beam if one knows how much plastic deformation has occurred. For example, for pure elastic
deformation the amount of bending force needed to produce a given amount of bending ("flexural stiffness") is proportional to the moment of inertia of
the (symmetric) beam cross section about the axis perpendicular to the applied force, i.e. the integral of the square of the distance (in the
direction of the applied force) to the center of gravity, taken over the entire cross section with respect to area. (The derivation of this is simple
enough: imagine the beam as being composed of parallel fibers. If it's bent into an arc, how much does each fiber need to stretch, and how does this
contribute to the overall bending force ("moment")?) One can find the force required for completely plastic deformation (i.e. plastic throughout the
entire cross section) similarly.
To wit, the moment of inertia of a circle of radius 1 is pi/4 (a somewhat hairy integral to do directly, use the "perpendicular axis theorem"),
whereas that of a square of the same cross sectional area along the axis parallel/perpendicular to the flats is (pi)^2/12, if I haven't screwed
anything up. Thus, for bars of equal length and weight the square bar is stiffer by a factor of pi/3, with the load applied along the flats, and so
the required load for the same (elastic) deflection will be approximately 92.7kg (the assumption being that the deflection is due primarily to
bending). Approximate solutions can be found similarly for the other cross sections. If plastic deformation is occurring, then I suspect that the
desired ratio will be somewhere between the elastic ratio, as above, and the ratio of force for completely plastic deformation (to get this,
approximately, use absolute value instead of the square in the formula for the moment of inertia).
|
|
|
math
Hazard to Others
  
Posts: 101
Registered: 21-7-2006
Member Is Offline
Mood: No Mood
|
|
thinking again, it may be needed a second moment of inertia
defined as
picture
where the area is perpendicular to the axis of bending. The bigger this moment of inertia is, the harder it is to bend or deform the beam, so one can
use proportion to find what is needed.
However it may not be that simple, as it would be too nice to consider that a circle of D diameter has an area that's 78.5% the one of a square with
same diameter, and hence the force required to bending one is 78.5% the one required to bend the other.
[Edited on 30-6-2008 by math]
|
|
|
franklyn
International Hazard
    
Posts: 3026
Registered: 30-5-2006
Location: Da Big Apple
Member Is Offline
Mood: No Mood
|
|
No there is no direct relation. In fact the cross sectional area of the material
can be reduced by for example fluting and the stiffness would be increased,
the principal reason for H and I beam forms. Similarly in building construction,
a segmented beam ( a sandwich of 2 X 12 individual planks interfaces oriented
vertically ) has greater bearing strength than the equivalent monolithic beam.
.
|
|
|
tumadre
Hazard to Others
  
Posts: 171
Registered: 10-5-2005
Member Is Offline
Mood: No Mood
|
|
| Quote: | Originally posted by math
However it may not be that simple, as it would be too nice to consider that a circle of D diameter has an area that's 78.5% the one of a square with
same diameter, and hence the force required to bending one is 78.5% the one required to bend the other.
[Edited on 30-6-2008 by math] |
"The ratio between the two forces is very close to the ratio of cross sectional area squared. .785^2 = .616" (i said that in the ninth post)
(Your data was 64.3 and 63.1% circle vs. square)
It's just a second order relationship. ignoring the differences in geometry. Comparing two circles would be closer to the square of the area ratio.
[Edited on 2-7-2008 by tumadre]
|
|
|